Squaring the Circle
August 21st, 2007
An exercise in contemplative geometry from Robert Lawlor’s Sacred Geometry, 1982.
“There are a number of diagrams in the literature of Sacred Geometry all related to the single idea known as ‘Squaring of the Circle’. This is a practice which seeks, with only the usual compass and straight-edge, to construct a square which is virtually equal in perimeter to the circumference of a given circle, or which is virtually equal in area to the area of a given circle. Because the circle is an incommensurable figure based on π, it is impossible to draw a square more than approximately equal to it. Nevertheless the Squaring of the Circle is of great importance to the geometer-cosmologist because for him the circle represents pure, unmanifest spirit-space, while the square represents the manifest and comprehensible world. When a near equality is drawn between the circle and the square, the infinite is able to express its dimensions or qualities through the finite” (p74).
Here I have drawn the former arrangement (perimeter equal to circumference):
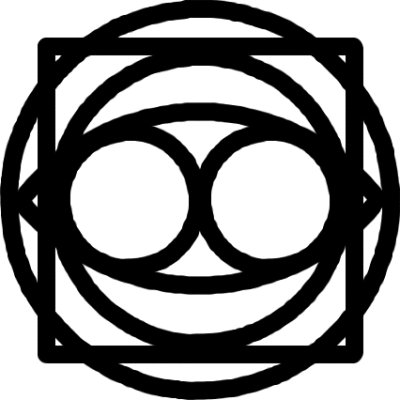
The derivation begins with an initial circle (within the square) of radius unity. Along its horizontal diameter are drawn two tangent circles, each with radius one half. Observe that the total circumference of the smaller circles equals the circumference of the initial circle, but the total area of the smaller circles is one half that of the initial circle: “One has become Two” (p73), an image of the primary duality, of yin-yang.
Next are drawn two arcs from the ends of the initial circle’s vertical diameter with radius tangent to the far sides of the smaller circles. This radius is φ, the golden ratio, dividing the vertical radius of the initial circle into the golden section of lengths 1/φ and 1/φ2. The two arcs meet to create a vesica that encloses the primary duality — the mouth of Ra, the Word, the vibrating string.
Around the initial circle is drawn a tangent square, with side 2, perimeter 8; and, finally, a large circle is drawn with diameter equal to the width of the vesica, 2√φ, giving a circumference of 2π√φ = 7.993, approximately equal to 8. The circle is squared.
August 22nd, 2007 at 8:40 am
A nice & instructive construction. A few comments are in order:
1) the passage from the ONE to the TWO always happens by MITOSIS, as it is witnessed innumerable times in the biological world: see
http://en.wikipedia.org/wiki/Mitosis
2) the WORD (logos), aka the number 3, is the MEDIATOR, i.e. the RATIO (it is not by chance that the word reason comes from the latin root ratio). This ratio is well represented by the number phi, the RATIO AUREA, or the eternal harmony inside duality.
3) out of the 3 comes the 4, the FOUR VISIBLE ELEMENTS, the SQUARE of directions (the fifth element being the original circle itself, itself an expansion of the central dot). The 4 externalized is the VISIBLE WORLD, represented by the outer circle. The fact that the square and the outer circle are indeed the same in perimeter, points to their fundamental identity.
A good Mandala for endless meditations….
August 23rd, 2007 at 7:19 pm
Mirco — 1, 2, 3, 4 (+1)…
From Lawlor’s original diagram I excluded 5 — a pentagon inscribed within the initial circle — in order to focus attention on the 4-sided objective.
But let’s not leave your finely presented progression hanging; for your mandalic meditations I include 5’s description here:
The pentagon “is the figure ascribed to the physical and vital aspects of man [i.e., Life], who, through the five senses, perceives the natural world and thus brings it into existence. The star pentagram formed by the diagonals within the pentagon symbolizes transformed or perfected humanity, because all the line segments of the star pentagram are derived from the Golden Proportion” (p75).
August 24th, 2007 at 8:31 am
I see…the pentagon, as the seal of the fifth element, is rightly inscribed in the ORIGINAL
circle.
It is the 1 AND the 5.
What Lawlor says is correct, albeit a bit abstract: the fifth seal indeed symbolizes the PERFECTED MAN, i.e. the first (and lower) degree of IMMORTAL.
I will not spoil other’s meditations on this remarkable construction, but I will add one more thing.
There are TWO kinds of pentagrams: one pointing UPWARD (lifting up the elements), and the other pointing DOWNWARD (the well known mark of the Dark One). When Man, passing beyond the impermanent material plane, reaches the world of the Force, the Path bifurcates….
October 21st, 2007 at 12:06 pm
[…] the alchemist performs the squaring the circle [see earlier post], thereby turning the two sexes into one. The motto repeats a saying of the ‘Rosarium’: […]
April 24th, 2008 at 5:37 am
Members of the WordPress community may like to see some truly ancient sacred geometry:
http://sarsen56.wordpress.com/solve-this/
June 2nd, 2008 at 2:15 am
A most interesting exercise.
I have made a sculpture of this construction,albeit in THREE DIMENSIONS.
http://ou8nrtist2.deviantart.com/art/Squaring-the-Circle-2-84483826
I have noticed a very subtle yet palpable vibratory resonation (for lack of a better or more rational term),the closer the two VOLUMES are to congruency…
February 18th, 2009 at 9:12 am
cool
February 14th, 2010 at 2:56 pm
[…] Figura Mentis, p307. C.f. Cusanus’s paradigmatic diagram and the mouth of Ra. […]
April 17th, 2011 at 4:52 am
sir , i have squared a circle exactly with a new value of pi. i will send my article.your postal address please.
rsj reddy
india
February 17th, 2012 at 11:39 am
[…] Figura Mentis, p307. C.f. Cusanus’s paradigmatic diagram and themouth of Ra. […]
February 16th, 2013 at 1:53 pm
New perspective on the geometry of a circle, once squared: http://aitnaru.org/thekingdom.html
August 1st, 2013 at 10:20 pm
Still running in squared circles, but the new “Pivotal Confirmation” corral offers out-of-the-box perspective on this “impossibility”: http://www.aitnaru.org/images/Pi_Corral.pdf
August 12th, 2017 at 1:23 am
To
Professors of the world
Sir,
Till now we have been accepting Exhaustion method of Archimedes as perfect and error – free in computing pi is less than 22/7. Hence pi is 3.14159265358.. But it is proved his method is wrong .
I would think this news would be discussed in the departmental seminar .
Regards
author
———- Forwarded message ———-
From: Mathematician Vitthal Jadhav
Date: Sun, Aug 6, 2017 at 9:31 PM
Subject: Re: 117 Method on Cosmic Pi
To: Sarva Jagannadha Reddy
Cc: bikasbikashchakraborty.math@yahoo.com
Mistakes in the #Archimedes #pi value computed by using Method of exhaustion.
(Issues with Traditional Pi (#?) )
—————————————————————————-
Assume circle, now draw the square inscribed in given circle,
the perimeter of square is clearly less than circumference,
Now if we increase side, draw regular hexagon with the given circle then difference between perimeter of hexagon & circumference will be reduced. Archimedes by using basic instrument like compass bisected each side of hexagon & obtained 12 point equally spaced on curved path of circle, join them & constructed regular dodecagon, in this way he continued this process. He calculated perimeter of regular polygon with 96 side & came to conclusion that the value of pi lies between
3 + 10/71 < ? < 3 + 10/70,
i.e. 3.140845 < ? infinity)
then delta tends to 0,
Now perimeter = n * (side of polygon i.e. AB)
Circumference = n * (Arc AB)
Error = n * ((Arc AB ) – (Side AB))
= n * delta
we clearly see, as n -> infinity , delta -> zero
but error doesn’t tend to zero as
it is “n * delta” becomes indeterminate
Archimedes method assumes error become insignificant.
This gives the reason – why cosmic pi = 3.146 given by Indian Professor R S Reddy (Sarva Jagannadha Reddy – We can call him as “PI Man” ) differ from traditional ? = 3.1459 approximately by 0.004
March 4th, 2018 at 11:57 pm
The comment of Vitthal Jadhav is based on a false statement with respect to the limiting value of the error term, and it is systematically flawed because he considers a lower bound for pi only and neglects the upper boundary. When asked about these problems, Vitthal Jadhav replied (https://www.researchgate.net/publication/319165096_Mistakes_in_Archimedes%27s_Method_of_Exhaustion_in_Computing_Pi_number_314159265358_by_Prof_Vitthal_Jadhav/comments):
>> J. M. Wagner , you are talking right. I already detected & accepted defects in my analysis. In my opinion it is not at all cosmic as it is not accurate value. Somewhere there is very minute error In R.S. Reddy’s analysis of Pi. For that the expert mathematician have to verify every step & assumption involved in RS Reddy’s method.
Here is whole conversion that occurred on this topic from my side:
**** Start of conversations *********
One more analysis that will really confuse & exactly contrary to previous, Respected Sir, I had not proven value of pi = 3.146446 is correct. Just shown as As n -> infinity, n * (delta x) will be in indeterminate form doesn’t mean computing area by dividing into infinite pieces gives wrong result. for x -> 0 , tan(x) / x is in indeterminate form but its limit exist & it is slightly greater than 1. Similarly, For x ->0, sin(x) / x = 1 (slightly less than 1) In this case, sin(x) can be represented as the function of x. sin (x) = (x) – (x^3 / 3!) + (x^5 / 5!) – (x^7 / 7!) +…. Clearly sin(x) is multiple of x ; sin (x) / x = 1 – x^2 / 3! + (x^4 / 5! ) Now, Area of n sided regular polygon inscribed in circle n * (1/2) r^2 sin(2?/n) = n* (1/2) * r^2 * (2?/n) as n -> infinity clearly it tends to ? * (r^2) that means error becomes insignificant as n-> infinity which is quite “contrary” result to previous. Now question is which one is correct ? *** <> Sorry for misinterpretation, But this result is not contrary to my previous analysis but it is consistent. In previous analysis, I showed that Error = n* (delta x) will be in indeterminate form as n -> infinity & delta -> zero. It doesn’t mean limit doesn’t exist. Also concluding n* (delta x) is significant or insignificant at this stage is “illogical”. Whether error become significant or insignificant can be seen by recent analysis i.e. Area of n sided regular polygon inscribed in circle n * (1/2) r^2 sin(2?/n) = n* (1/2) * r^2 * (2?/n) as n -> infinity clearly it tends to ? * (r^2) Yes, error become insignificant as n -> infinity. Also by Inscribing regular polygon in the circle, increasing n to infinity even if we won’t get accurate value of pi up to all decimal place, that doesn’t mean it won’t gives accurate values at least up to five decimal places. ****** End of conversion *********** <<
May 6th, 2018 at 6:02 pm
Squaring the circle with equal areas:
Squaring the circle involves creating a circle with a circumference equal to the perimeter of a square. The correct word creating a circle that has a circumference that is equal in measure to the perimeter of a square is Rectifying the circle. The term Rectifying the circle is used to prevent confusion with the creation of a circle and a square with the same surface area because some people only think that the phrase squaring the circle only applies to creating a circle and a square with the same surface area and in many social circles of mathematicians squaring the circle is used only to describe the creation of a circle and a square with the same surface area. Also squaring the circle can involve creating a circle and a square with equal areas or approximate equal areas. Squaring the circle can also include harmonious relationships such as the part of the square that intersects the circle’s circumference can be similar to the radius of the circle or the same as the radius of the circle or equal to half of the square’s edge length. Squaring the circle with the area of the square being equal to the area of the circle usually cannot be achieved with 100% accuracy because traditional Pi 3.141592653589793 has been proven to be Transcendental in addition to being irrational. Traditional Pi 3.141592653589793 is Transcendental because Traditional Pi 3.141592653589793 does not fit any polynomial equations. Squaring the circle becomes possible and easy after traditional Pi 3.141592653589793 has been rejected and replaced with other values of Pi that are NOT transcendental. Golden Pi = 3.144605511029693 is irrational but Golden Pi is NOT transcendental because Golden Pi = 3.144605511029693 is the only value of Pi that fits the following polynomial equation:
4th dimensional equation/polynomial for Golden Pi = 3.144605511029693 (x4 + 16×2 – 256 = 0).
(https://www.tiger-algebra.com/drill/x~4-16x~2-256=0/)
Pi Math Proof: http://measuringpisquaringphi.com/pi-math-proof/
A polynomial is an expression consisting of variables (or indeterminates) and coefficients, that involves only the operations of addition, subtraction, multiplication, and non-negative integer exponents of variables. An example of a polynomial of a single indeterminate x is x2 ? 4x + 7. An example in three variables is x3 + 2xyz2 ? yz + 1.
Polynomials appear in a wide variety of areas of mathematics and science. For example, they are used to form polynomial equations, which encode a wide range of problems, from elementary word problems to complicated problems in the sciences; they are used to define polynomial functions, which appear in settings ranging from basic chemistry and physics to economics and social science; they are used in calculus and numerical analysis to approximate other functions. In advanced mathematics, polynomials are used to construct polynomial rings and algebraic varieties, central concepts in algebra and algebraic geometry.
Both Golden Pi = 3.144605511029693 and Pi accepted as 22 divided by 7 = 3.142857142857143 can be used to create a circle and a square with equal areas of measure involving 100% accuracy. 2 examples of creating a circle and a square with 100% accuracy:
Example 1 creating a circle and a square with equal areas involving 100% accuracy with Golden Pi = 3.144605511029693 :
My question is it possible to create a circle with a surface area of 106 equal units because if we can create a circle with a surface area of 106 equal units of measure then we can also create a square with a surface area of 106 equal units of measure by creating a scalene right triangle with the second longest edge length as 9 equal units of measure taken from the diameter of the circle that has a surface area of 106 equal units of measure, while the shortest length of the scalene right triangle has 5 equal units of measure. The hypotenuse of a scalene right triangle with the second longest length as 9 equal units of measure and the shortest length of the scalene right triangle as 5 equal units of measure is equal in measure to the width of a square that has a surface area of 106 equal units of measure. We can use the theorem of Pythagoras: to prove that the square with a width equal to the longest length of the scalene right triangle also called the hypotenuse also has 106 equal units of measure.
Area of circle = 106.
Rational measure for the diameter of circle = 11.62.
Irrational measure for the diameter of the circle = 11.61180790611399 according to Golden Pi = 3.144605511029693.
Irrational measure for the diameter of the circle = 11.61180790611399 divided by the width of the square the square root of 106 = the square root of the Golden root = 1.127838485561683.
The Golden root = 1.272019649514069.The Golden root = 1.272019649514069 is the square root of Golden ratio of Cosine (36) multiplied by 2 = 1.618033988749895.
Irrational measure for the circumference of the circle = 36.514555134584213 according to Golden Pi = 3.144605511029693.
Square root of Golden Pi = 3.144605511029693 = 1.773303558624324
9 squared = 81.
5 squared = 25.
81 + 25 = 106.
Most values of Pi can confirm that if a circle has a rational measure for the diameter as 11.62 equal units of measure then the surface area of the circle with a rational measure for the diameter of 11.62 equal units of measure is 106 equal units of measure.
“The ancient Egyptian square root of Pi rectangle and the ancient Egyptian square root for the Golden root rectangle and also the Pythagorean theorem”: https://en.wikipedia.org/wiki/Pythagorean_theorem
Example 2 creating a circle and a square with equal areas involving 100% accuracy with 22 divided by 7 = 3.142857142857143 as Pi.
Is it possible to create a circle with a surface area of 154 equal units because if we can create a circle with a surface area of 154 equal units of measure then we can also create a square with a surface area of 154 equal units of measure by creating a scalene triangle with the second longest edge length as 12 equal units of measure taken from the diameter of the circle that has a surface area of 154 equal units of measure, while the shortest length of the scalene triangle has 3 plus 1 equal units of measure. The hypotenuse of a scalene right triangle with the second longest length as 12 equal units of measure and the shortest length of the scalene triangle as 3 plus 1 equal units of measure is equal in measure to the width of a square that has a surface area of 154 equal units of measure. We can use the theorem of Pythagoras to prove that the square with a width equal to the longest length of the scalene right triangle also called the hypotenuse also has a surface area of 154 equal units of measure.
Area of circle = 154.
Diameter of circle = 14.
Circumference of circle = 44.
Ancient Egyptian Pi = 22 divided by 7 = 3.142857142857143.
12 squared = 144.
3 squared = 9.
1 squared = 1
144 + 9 + 1 = 154.
“The ancient Egyptian square root of Pi rectangle and the ancient Egyptian square root for the Golden root rectangle and also the Pythagorean theorem”:
A square with a surface area of 154 equal units of measure can be created if the second longest edge length of a scalene right triangle has 12 equal units of measure while the shortest edge length of the scalene right triangle has 3 plus 1 equal units of measure. According to the Pythagorean theorem if a square has a width that is equal to the hypotenuse of a scalene right triangle that has its second longest edge length as 12 equal units of measure while the shortest edge length for the scalene right triangle has 3 plus 1 equal units of measure then the surface area of the square that has a width equal to the measure of the hypotenuse for the scalene right triangle that has its second longest edge length as 12 while its shortest edge length is 3 plus 1 equal units of measure is 154 equal units of measure. If the width of the square that has a surface area of 154 equal units of measure is then used as the longer length of a square root of ancient Egyptian Pi = 1.772810520855837 rectangle then a circle can be created with the shorter edge length of the square root of ancient Egyptian Pi = 1.772810520855837 rectangle being equal in measure to the radius of the circle with a surface area equal to the surface area of the square that has a surface are of 154 equal units of measure. According to ancient Egyptian Pi = 3.142857142857143 if the radius of a circle has 7 equal units of measure then the surface are of the circle is 154 equal units of measure. The measuring angles for a square root of ancient Egyptian Pi = 1.772810520855837 rectangle are 60.57369496075449 degrees and 29.42630503924551 degrees. 60.57369496075449 degrees can be gained when the square root of ancient Egyptian Pi = 1.772810520855837 is applied to the inverse of Tangent in Trigonometry. 29.4263050392455 degrees can be gained when the ratio 0.564076074817766 is applied to the inverse of Tangent in Trigonometry. If a circle with a diameter of 14 equal units of measure has already been created so that the surface area of the circle can have 154 equal units of measure according to ancient Egyptian Pi = 3.142857142857143 and the desire is to have a square that also has a surface area equal to the circle’s surface area of 154 equal units of measure then a solution is to add 1 quarter of the circle’s circumference that is 11 to the diameter of the circle with 14 equal units of measure and at the division point where 14 is subtracted from the diameter line of 25 equal units of measure draw right angles that can touch the circumference of a circle or a semi-circle if the diameter of 25 equal units of measure is divided into 2 halves. A rectangle with its longest length as 14 while its second longest length is the square root of 154 has the ratio for the square root of the Golden root = 1.127838485561682 approximated to 1.128152149635533. 1.128152149635533 is the square root of 1.272727272727273. 4 divided by 1.272727272727273 is ancient Egyptian Pi = 3.142857142857143. So the longer length of the ancient Egyptian square root for the Golden root = 1.128152149635533 rectangle is 14, the diameter of the circle with a surface area of 154, while the shorter length of the ancient Egyptian square root for the Golden root = 1.128152149635533 rectangle is the square root of 154 = 12.40967364599086, the width of the square.
1.128152149635532 squared is 1.272727272727272 and 1.272727272727272 squared is the Golden ratio approximated to 1.619834710743799. The ancient Egyptian square root for the Golden root = 1.128152149635532 is important.
Area of circle = 154.
Diameter of circle = 14.
Circumference of circle = 44.
Pi as 22 divided by 7 = 3.142857142857143.
2 methods for calculating the surface area of a circle
Method 1 = Radius of circle = 7 .7 squared = 49. 49 multiplied by Pi as 22 divided by 7 = 3.142857142857143 = 154.
The surface area of a circle that has 154 equal units of measure can also be calculated if the diameter of the circle is divided by the Square root of Phi = 1.272019649514069 approximated to 14 divided by 11 = 1.272727272727273 resulting in 1 quarter of the circle’s circumference 11 and then half the circumference of the circle 22 is then multiplied by 14 the measure for the diameter of the circle and then the result of multiplying half the circumference of the circle 22 by the measure for the diameter of the circle 14 is divided in 2 resulting in the measure for the surface area of the circle = 154.
A square with a surface area of 154 equal units of measure can be created according to the Pythagorean theorem through the following formula:
12 squared = 144.
3 squared = 9.
1 squared = 1.
144 + 9 + 1 = 154.
The edge of the square with a edge length of 12 is placed on the same angle and line as the extended hypotenuse of the right triangle that has its second longest edge length as 3 while the shortest edge of the right triangle is 1. According to the Pythagorean theorem a right triangle that has its second longest edge length as 3 while the shortest edge of the right triangle is 1 has the hypotenuse equal to the square root of 10.)
Quadrature of the circle constants again
Phi = Cosine (36) multiplied by 2 = 1.618033988749895.
The square root of Phi = 1.272019649514069.
1.272019649514069 squared = 1.618033988749895.
The square root of the square root of Phi = 1.127838485561682.
1.127838485561682 squared = 1.272019649514069.
The true value of Pi = 4 divided by 1.272019649514069 = 3.144605511029693144.
The square root of Pi = 2 divided by 1.127838485561682 = 1.773303558624324.
1.773303558624324 squared = 3.144605511029693144.
May 24th, 2018 at 9:06 am
“Constructing the irrational ratio Pi on a straight line”:
Traditional Pi 3.141592653589793 has been proven to be Transcendental in addition to being irrational. Traditional Pi 3.141592653589793 is Transcendental because Traditional Pi 3.141592653589793 does not fit any polynomial equations. Squaring the circle with equal surface areas becomes possible and easy after traditional Pi 3.141592653589793 has been rejected and replaced with other values of Pi that are NOT transcendental. Golden Pi = 3.144605511029693 is irrational but Golden Pi is NOT transcendental because Golden Pi = 3.144605511029693 is the only value of Pi that fits the following polynomial equation:
4th dimensional equation/polynomial for Golden Pi = 3.144605511029693 (x4 + 16×2 – 256 = 0). (https://www.tiger-algebra.com/drill/x~4-16x~2-256=0/
A polynomial is an expression consisting of variables (or indeterminates) and coefficients, that involves only the operations of addition, subtraction, multiplication, and non-negative integer exponents of variables. An example of a polynomial of a single indeterminate x is x2 ? 4x + 7. An example in three variables is x3 + 2xyz2 ? yz + 1.
Polynomials appear in a wide variety of areas of mathematics and science. For example, they are used to form polynomial equations, which encode a wide range of problems, from elementary word problems to complicated problems in the sciences; they are used to define polynomial functions, which appear in settings ranging from basic chemistry and physics to economics and social science; they are used in calculus and numerical analysis to approximate other functions. In advanced mathematics, polynomials are used to construct polynomial rings and algebraic varieties, central concepts in algebra and algebraic geometry.
Please remember that if the second longest edge length of a Kepler right scalene triangle is used as the measure for the diameter of a circle then the shortest edge length of the Kepler right triangle is equal to 1 quarter of the circle’s circumference. The longer length of the construction of the irrational ratio Pi on a straight line must be divided into 4 equal sections. The second longest edge length of a Kepler right scalene triangle that has its shortest edge length equal in measure to 1 quarter of the larger measure for the construction of the irrational ratio Pi on a straight line must be added to line that has already been dived into 4 equal sections. Alternatively the construction of the irrational ratio Pi on a straight line can also start with the second longest edge length of the Kepler right scalene triangle plus the shortest edge length of the Kepler right scalene triangle being multiplied 4 equal times and then added to the second longest edge length of the Kepler right scalene triangle.
A given line can divided into the irrational ratio Pi if 2 of the 3 lengths of the scalene triangle are first used to construct the irrational ratio Pi on a straight line and the given desired line is 1 of the 3 lengths of the scalene triangle that has not been divided yet. The divisions of the 2 other lengths of the scalene triangle into the ratio Pi can then be transferred on to the given desired line resulting in the given desired line being divided into the irrational ratio Pi.
The ratio Golden Pi = 3.144605511029693 can also be constructed on a straight line if 1 quarter of the second longest edge length of a Kepler right triangle is added to the measure for the shortest edge length of a Kepler right triangle. The measure for the shortest edge length of a Kepler right triangle divided by 1 quarter of the measure for the second longest edge length of a Kepler right triangle = Golden Pi = 3.144605511029693.
The ratio half of Pi can be constructed if an isosceles triangle that is made from 2 Kepler right triangles is created and the base width of the isosceles triangle that is made from 2 kepler right triangles
Is divided by the height of the isosceles triangle that is made from 2 Kepler right triangles.
Constructing a rectangle with the irrational ratio Pi:
If a Kepler right triangle is created and a rectangle is also created that has its longest edge length equal to the shortest edge length of a Kepler right triangle while the shorter edge of the rectangle is equal to 1 quarter of the second longest edge length of the Kepler right triangle then the ratio that can be gained if the longer edge of this rectangle that has its longer length equal to the shortest edge length of a Kepler right triangle is divided by the shorter edge length of this rectangle that is equal to 1 quarter of the second longest edge length of a Kepler right triangle is Golden Pi = 3.144605511029693.
The ratio 3.299779359290302 is the square root of the ratio 10.888543819998315. The ratio 3.299779359290302 applies to the diagonal of a Golden Pi = 3.144605511029693 rectangle divided by the shorter edge length of a Golden Pi rectangle. If a right triangle is created that produces the ratio Golden Pi = 3.144605511029693 when the second longest edge length of the right triangle is divided by the shortest edge length of the right triangle then the result of dividing the measure for the hypotenuse of the right triangle by the shortest edge length of the right triangle is the ratio 3.299779359290302.
The ratio 3.299779359290302 can be gained also if the square root of 17.618033988749895 is divided by the square root of the Golden ratio = 1.272019649514069. Please remember that the ratio 17.618033988749895 is equal to the Golden ratio of Cosine (36) multiplied by 2 = 1.618033988749895 plus 16. The ratio 3.299779359290302 can also be gained if the result of adding Golden Pi squared = 9.888543819998317 plus 1 squared is applied to square root according to the Pythagorean theorem. If the longer measure for a Golden Pi = 3.144605511029693 rectangle is 4 then the shorter measure for the Golden Pi = 3.144605511029693 rectangle is equal to the square root of the Golden ratio = 1.272019649514069,while the measure for the diagonal of the Golden Pi = 3.144605511029693 rectangle is equal to the square root of 17.618033988749895. Please remember again that the ratio 17.618033988749895 is equal to the Golden ratio of Cosine (36) multiplied by 2 = 1.618033988749895 plus 16.
“Gaining the ratio Pi by from squaring the circle with equal perimeters. The creation of a circle with a circumference equal in measure to the perimeter of a square”:
If a circle is created with a circumference equal in measure to the perimeter of a square then the ratio Pi can be gained if either the circumference of the circle or the perimeter of the square is divided by the diameter of the circle.
If a circle is created with a circumference equal in measure to the perimeter of a square then the ratio Pi can be gained if either the half of the circumference of the circle or the half of the perimeter of the square is divided by the radius of the circle. If the diameter of the circle or the radius of the circle is the second longest edge length of a Kepler right triangle, while the shortest edge length of the Kepler right triangle is equal to either 1 quarter of the circle’s circumference or one 8th of the circle’s circumference then the correct measure for the diameter of the circle can be known if the Pythagorean theorem is applied to all the edges of the Kepler right triangle. :
“Constructing Pi by placing the circumference of a circle on a straight line”:
Pi can easily be constructed by placing the circumference of a circle on a straight line next to the diameter of the circle. The circumference of a circle does NOT have to be curved. The circumference of a circle can also be a straight line. If an individual already knows how to divide the circumference of a circle into 12 equal parts by using compass and straight edge then that individual can construct the ratio Pi on a straight line by simply placing the 12 sections of the circle’s circumference on a straight line next to the diameter of the circle. The real value of Pi is NOT 3.141592653589793. The real value of Pi = 3.144605511029693144.
https://m.facebook.com/TheRealNumberPi/
http://www.measuringpisquaringphi.com
Download for free and keep and read The book of Phi volume 8: The true value of Pi = 3.144, by Mathematician and author Jain 108: https://lists.gnu.org/archive/html/help-octave/2016-07/pdf1s8_jmqrL6.pdf
(https://www.tiger-algebra.com/drill/x~4-16x~2-256=0/)
Kepler right triangle: https://www.goldennumber.net/triangles/
https://en.wikipedia.org/wiki/File:Kepler_Triangle_Construction.svg
July 1st, 2020 at 1:03 am
I send you my easy way of squaring the circle regarding to Phi with two squares, and compas to find the root of Pi / 2
http://fermancebo.com/Squaring_regarding_Phi.html